ICF-like mesh in four quadrants optimized by four ETHOS strategies

The Enabling Technologies for High-Order Simulations (ETHOS) project performs research of fundamental mathematical technologies for next-generation high-order simulations algorithms. The current focus is on advancing the theoretical understanding and practical utility of unstructured meshes with arbitrarily high-order curvilinear elements by researching key challenges associated with high-order mesh quality optimization and simulation-driven adaptivity.

With support from the Applied Mathematics research program in the Department of Energy (DOE) Office of Science, the ETHOS team performs fundamental research that is broadly applicable to many high-order simulation approaches, including high-order finite elements and tightly coupled arbitrary Lagrangian-Eulerian (ALE) simulations of interest to the DOE. Our research builds on the Target-Matrix Optimization Paradigm (TMOP) and nonlinear variational minimization to develop new node-movement strategies for high-order mesh quality optimization and adaptation in both purely geometric settings, as well as in the context of a given physical simulation.

Project summary

application in a practical ALE simulation

High-order meshes are difficult to control

  • High-order simulations rely on high-order meshes, and bad mesh quality leads to small time steps and applications failures.
  • In addition to good geometric quality, applications require high-order meshes to adapt to dynamic simulation features.

Our solution: Develop theory that rigorously defines high-order mesh quality

  • We have extended the Target Matrix Optimization Paradigm (TMOP) by Knupp to high-order meshes, defining pointwise quality based on sub-zonal information, and optimizing the mesh node positions with respect to an aggregated quality measure. The TMOP variational minimization technique is based on optimizing an objective function that depends on a quality metric measuring the difference between the current and target geometric parameters of the mesh.
  • We are currently exploring (nonlinear) solvers for the global optimization problem, incorporating research in constrained optimization, linear and nonlinear solvers, and preconditioners.
  • We are developing the TMOP-based variational formulation for the upcoming exascale architectures.
  • We are also interested in mesh generation, surface optimization, hrp-refinement, interface fitting, and more.

Impact: Mesh optimization is relevant to a wide range of applications. Some innovations under the ETHOS project include:

  • Target construction: Methods for systematic generation of geometric target parameter values (size, aspect-ratio, skew, and orientation) that vary over mesh elements or sample points. Permits flexible and precise control over essential geometric quality features of mesh, tailored to any specific application.
  • Mesh quality metrics: New polyconvex mesh quality metrics that allow control of one or more geometric parameters (size, aspect-ratio, skew, and/or orientation). Asymptotically balanced compound metrics that automatically balance mesh quality in terms of shape and size. Worst case quality improvement metrics to simultaneously untangle the mesh and decrease worst element quality.
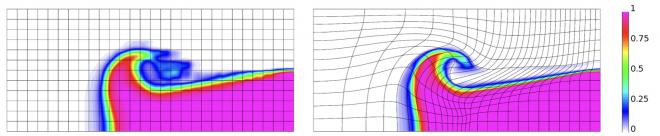
  • Simulation-driven r-adaptivity: Simulation-based adaptivity to optimize meshes during runtime using the solution of the PDE. This approach has already demonstrated significant impact in improving solution accuracy and computational efficiency for ALE hydrodynamics.
  • hr-adaptivity: TMOP-based energy estimators to augment r-adaptivity with anisotropic mesh refinement. hr-adaptivity is crucial when only r-adaptivity cannot meet the desired shape and size adaptivity goals.
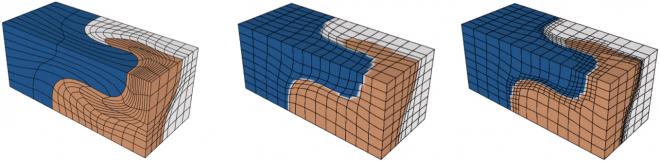
  • Surface fitting: Penalty-based formulation to align a subset of the mesh nodes to surfaces prescribed as the level-set of a discrete function. This approach is used to take a given mesh and morph it to obtain a body-fitted high-order mesh.
  • rp-adaptivity: Surface fitting augmented with p-adaptivity such that high-order elements are only used near curvilinear surfaces and low-order elements are used elsewhere. rp-adaptivity allows us to obtain mixed-order meshes with same geometrical accuracy as a uniform-order mesh but at a much lower computational cost. 
  • Adaptive node limiting: Penalty-based formulation to optimize the geometric quality of the mesh while minimizing the change associated with a discrete function defined on the original mesh. This functionality was inspired by multi-material applications where it is crucial to preserve material mass for each zone while optimizing the mesh quality.
  • GPU-oriented mesh optimization: Tensor factorization and matrix-free approach for TMOP-based algorithms results in orders of 20x speed-up for high-order mesh optimization in ALE hydrodynamics.
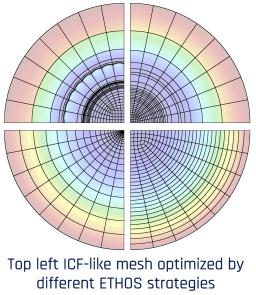
  • TMOP-based adaptivity has been used for a wide variety of applications ranging from inertial confinement fusion, where symmetry preservation is important, to tokamak magnetohydrodynamics, where mesh alignment with the magnetic fields helps accelerate the convergence of the solution.
  • The ETHOS algorithms are freely available on the MFEM website.

Team

Software

MFEM logo
  • Many of the high-order mesh optimization algorithms developed in the ETHOS project are freely available in a user-friendly form in the MFEM finite element library.
  • See in particular the Mesh Optimizer miniapp in the miniapps/meshing directory and the TMOP sources in the fem directory.

Publications

Presentations

Gallery

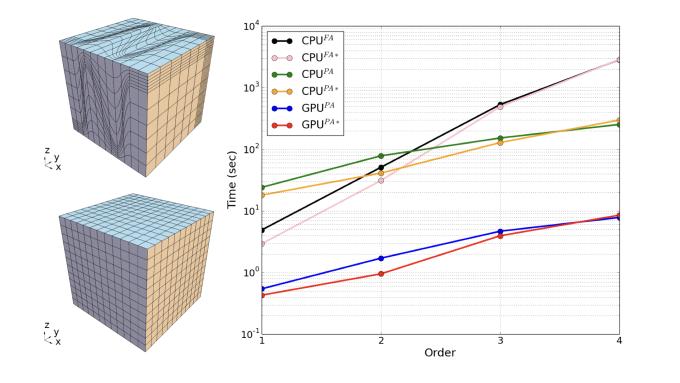
  • Benchmark featuring Kershaw meshes shows that finite element partial assembly on GPUs leads to significant speed-up (30-40x) in comparison to full assembly on CPUs. [paper]
(Top left) A Fourth-order Kershaw transformed mesh (bottom left) optimized using TMOP for high-order meshes. (Right) Comparison of time to solution for different approaches: PA represents partial assembly, FA represents full assembly, and (*) represents use of a preconditioner. 
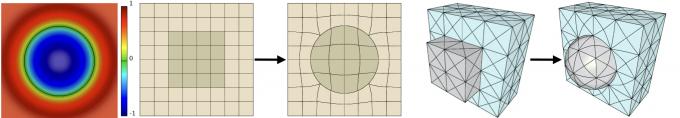
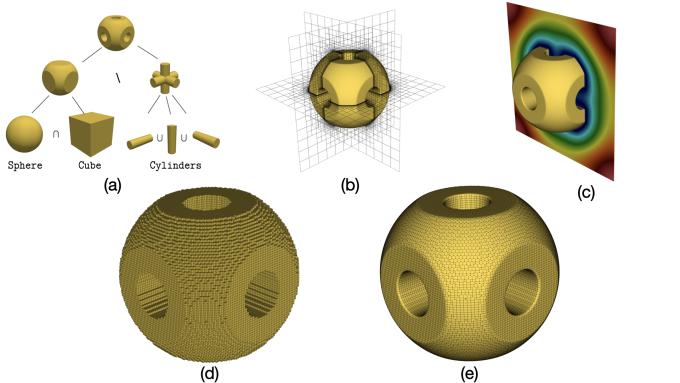
  • Boundary and interface alignment to surfaces prescribed as the zero level-set of a discrete function [paper1, paper2].
Circular interface represented using a level-set function and a Quadrilateral and a Tetrahedral mesh optimized to align to the target surface.
(a) CSG tree for a curvilinear 3D domain. (b) Adaptively refined mesh used to represent the domain of interest and (c) corresponding level-set function extracted using a distance function. (d) Easy-to-generate Cartesian aligned mesh trimmed and (e) optimized to fit to the target surface.
Topology optimization with conformal meshes to maximize beam stiffness under a downward force on the right wall. TMOP-based approach is used at each topology optimization iteration to obtain a boundary-fitted mesh. Click here for animation.
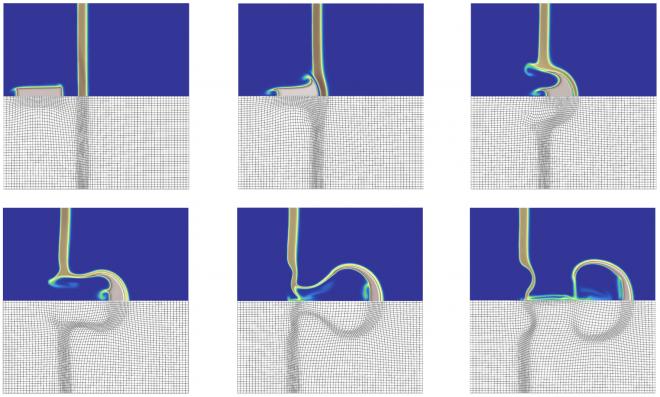
  • Simulation-driven r-adaptivity [paper]
Size and aspect-ratio based adaptivity using a material indicator function defined on a Cartesian mesh.
r-adaptivity for the gas impact problem. [movie]
Comparison of a cartesian mesh and an optimized mesh for the triple-point problem. r-adaptivity using the solution at runtime enables mesh alignment with material interfaces which reduces numerical discretization error.
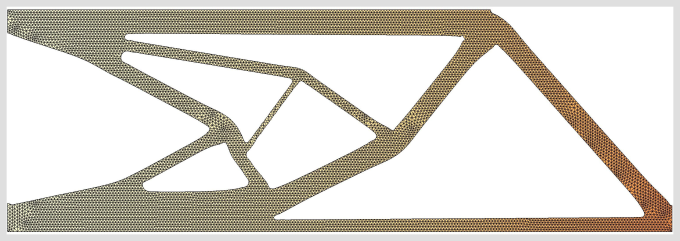
  • Comparison of a mesh obtained from multi-material ALE hydrodynamics calculation in Laghos with r-adaptivity and hr-adaptivity meshes. [paper]
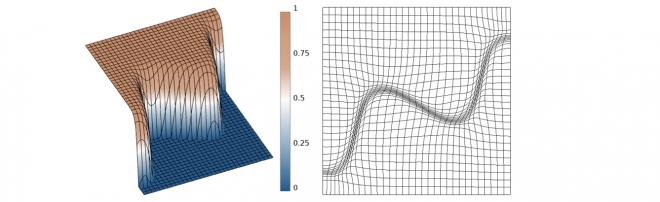
  • Some fun mesh optimization using images. The meshes shown below are colored by element sizes.